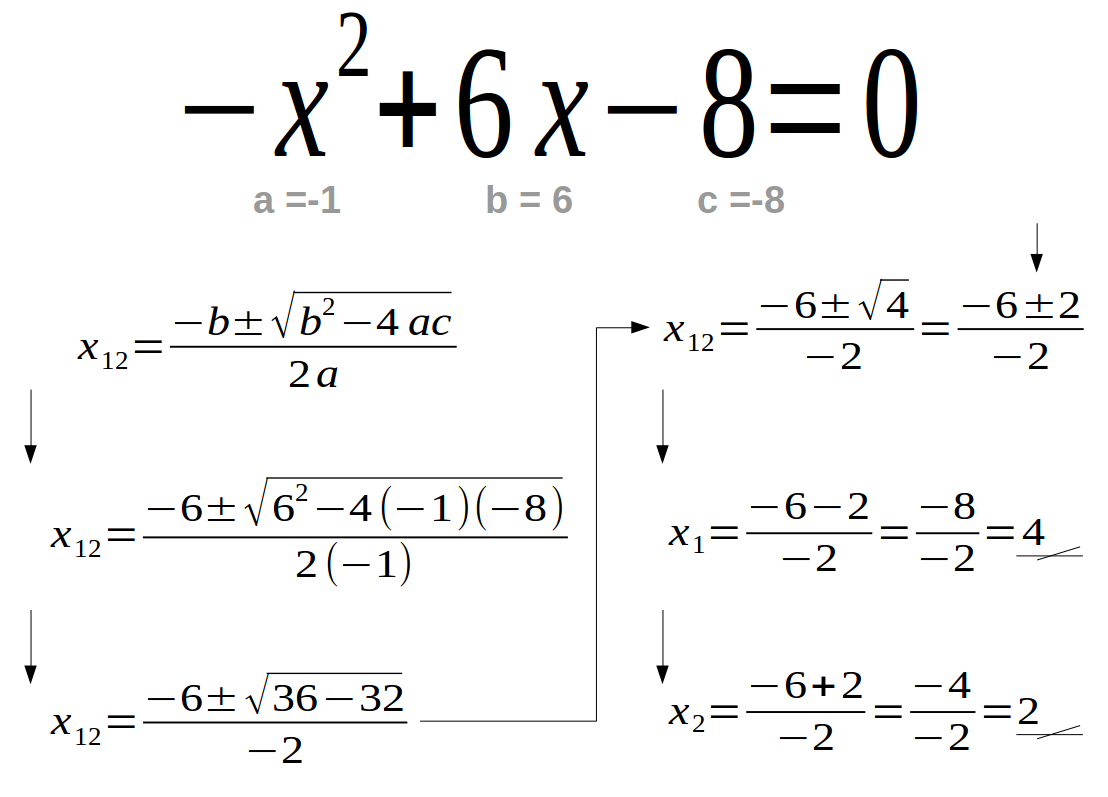Imagine trying to predict the trajectory of a basketball throw, or calculate the time it takes for an investment to double. These scenarios, and countless others, can be understood and solved using the power of mathematical equations. Specifically, first and second-degree equations, often encountered in algebra, provide a framework for unraveling these mysteries and many more.
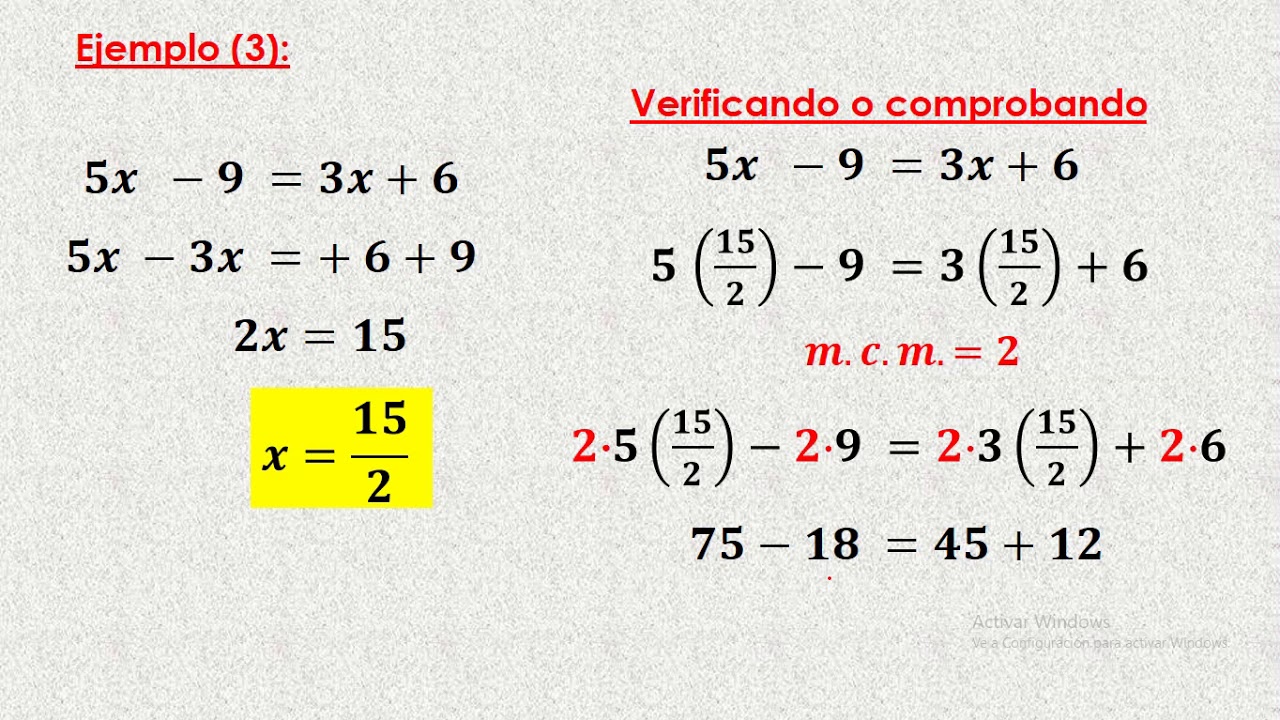
First-degree equations, also known as linear equations, involve finding the value of a single unknown variable. They are the building blocks of algebra, representing relationships like a straight line on a graph. Second-degree equations, or quadratic equations, introduce a higher level of complexity. They involve finding the values of variables raised to the power of two, often representing parabolic curves on a graph.
The history of these equations stretches back centuries, with contributions from ancient civilizations like the Babylonians and Egyptians. These early mathematicians developed methods for solving specific types of equations, laying the foundation for the more generalized approaches we use today.
The importance of first and second-degree equations extends far beyond the classroom. They form the backbone of numerous fields, including physics, engineering, computer science, economics, and even art. Understanding these equations empowers us to model and solve problems related to motion, forces, optimization, financial growth, and countless other real-world phenomena.
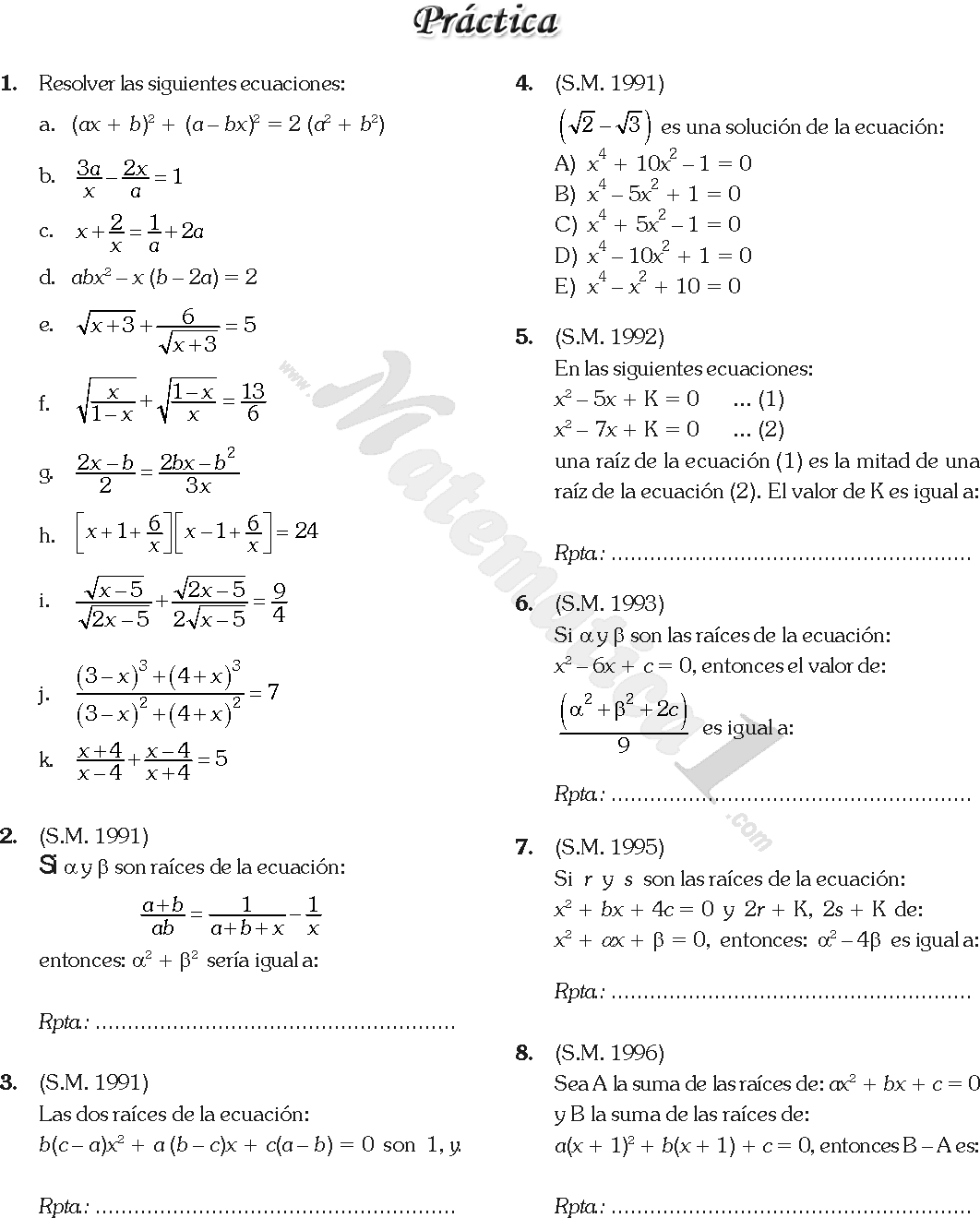
One of the main challenges in dealing with these equations is recognizing the appropriate type of equation for a given problem. Is it a linear relationship best described by a first-degree equation? Or does the problem involve a squared term, indicating the need for a quadratic equation? Accurately identifying the type of equation is crucial for selecting the correct methods for solving it.
Let's delve into some definitions and examples to clarify these concepts. A simple first-degree equation might look like this: 2x + 5 = 11. Solving for 'x' involves isolating the variable on one side of the equation, which in this case gives us x = 3. A second-degree equation, on the other hand, would include a squared term, like this: x² + 4x - 12 = 0. Solving for 'x' in this case requires different techniques, such as factoring or using the quadratic formula.
Now, let's explore the benefits of mastering these equations. First, they enhance problem-solving skills. By providing a structured approach to representing and manipulating relationships, these equations enable us to break down complex problems into smaller, more manageable steps.
Second, understanding these equations opens doors to a wide range of career paths. As mentioned earlier, fields from engineering to economics rely heavily on mathematical modeling using these equations. A strong foundation in algebra is often a prerequisite for pursuing higher education and careers in these areas.
Finally, mastering first and second-degree equations empowers us to make more informed decisions in our daily lives. Whether it's calculating the best interest rate for a loan or determining the optimal dimensions for a garden fence, these equations provide the tools for making informed choices.
In conclusion, while first and second-degree equations might initially seem like abstract mathematical concepts, their significance in our world is undeniable. From the trajectories of objects to the growth of investments, these equations underpin our understanding of countless phenomena. Mastering these equations not only strengthens our analytical abilities but also opens doors to a world of opportunities in diverse fields. So, embrace the power of algebra and unlock the solutions to the problems that surround us.
Making bing your default search a comprehensive guide
Sending holiday wishes via email a guide to festive digital greetings
The allure and illusion of the bad boys ride or die trope
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On
ecuaciones de primer grado y segundo grado - Khao Tick On





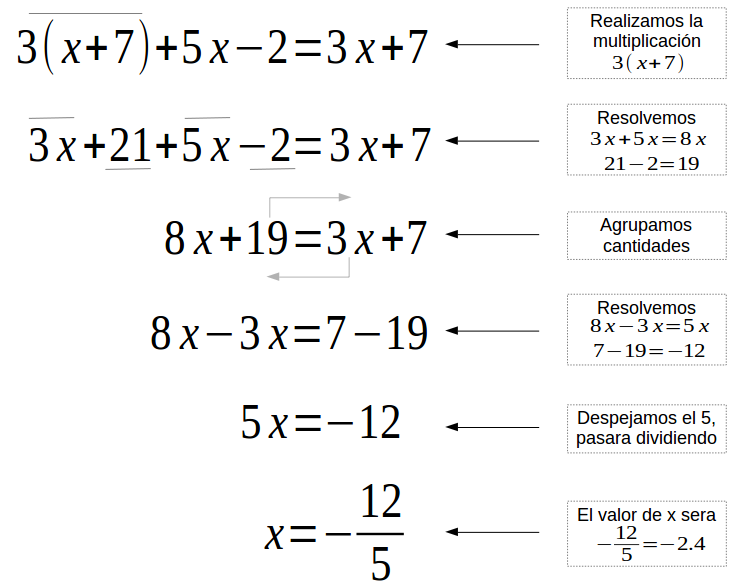



.gif)