Unlocking the Power of Triangles: Your Guide to Conquering Trigonometry Problems

02 Jun 2024
us
Ann

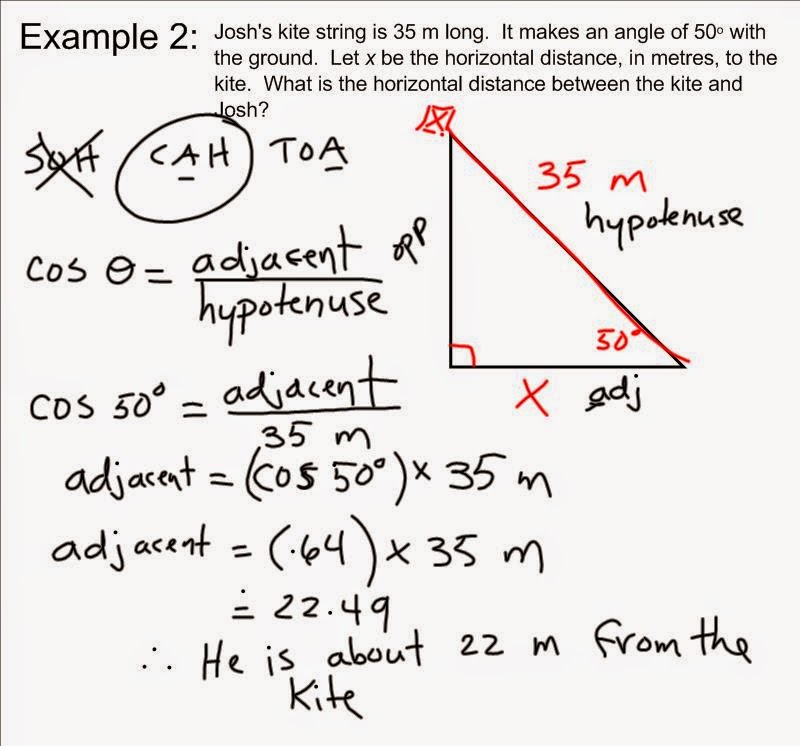
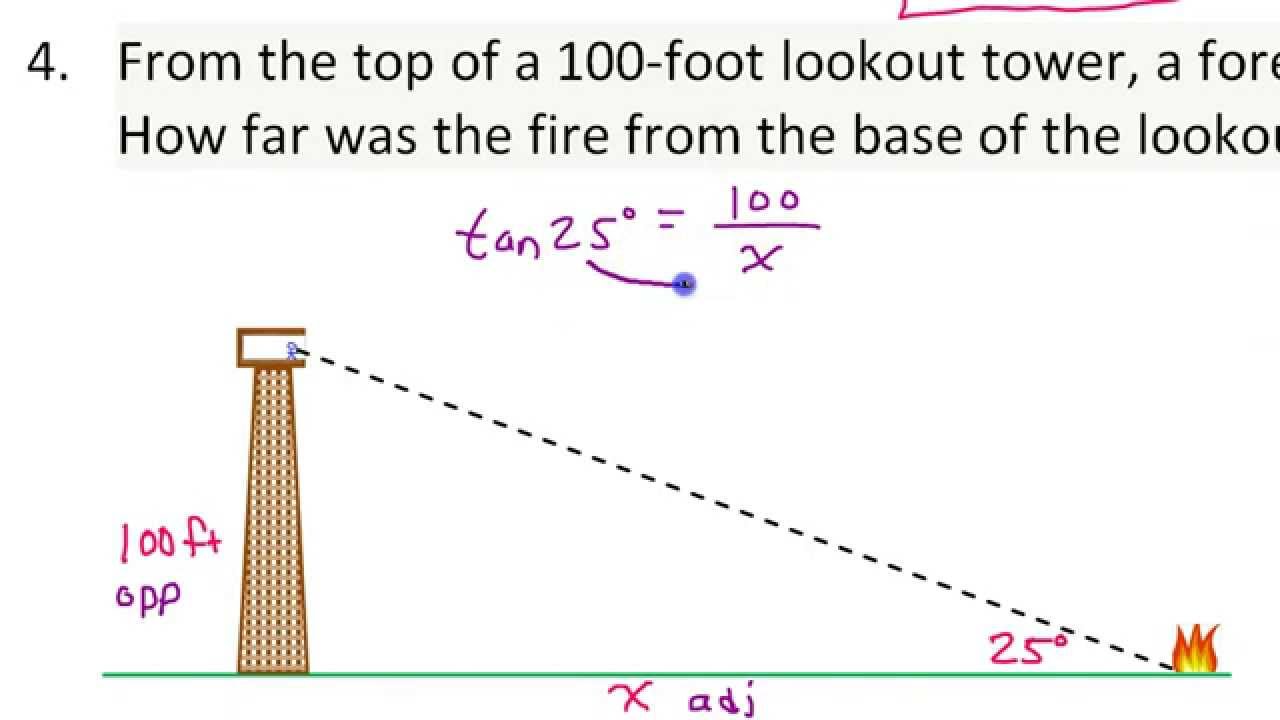
Have you ever gazed upon a towering skyscraper or a gracefully arched bridge and wondered about the mathematical wizardry behind their construction? Or perhaps you've been puzzled by the intricate dance of shadows and sunlight, wondering how to predict their movements? The answer, my friend, lies in the fascinating world of trigonometry.

Trigonometry, often perceived as a daunting realm of sines, cosines, and tangents, is essentially the study of triangles and the relationships between their sides and angles. While it may seem like an abstract mathematical concept, its applications are incredibly practical and far-reaching, underpinning fields like architecture, engineering, physics, and even music.

Mastering trigonometry problems is not about memorizing endless formulas but about understanding the fundamental principles and applying them strategically. This journey begins with grasping the core trigonometric functions – sine, cosine, and tangent – which describe the ratios between the sides of a right-angled triangle. From these building blocks, we can then explore more complex identities and theorems, unlocking the tools to solve a vast array of problems.

Imagine being able to calculate the height of a tree without climbing it, simply by measuring its shadow and the angle of the sun. Or picture yourself confidently navigating through unfamiliar territory, using your knowledge of bearings and distances. These are just a glimpse of the empowering capabilities that trigonometry offers.

Throughout history, civilizations have relied on trigonometry to solve practical problems. Ancient Egyptians used it to survey land and construct pyramids, while astronomers employed its principles to map the stars and predict celestial events. Today, trigonometry continues to be an indispensable tool in numerous fields, highlighting its enduring relevance and power.

Advantages and Disadvantages of Learning Trigonometry

While learning trigonometry can be incredibly rewarding, it's essential to approach it with a clear understanding of both its advantages and potential challenges.

Advantages	Disadvantages
Enhances problem-solving skills	Can be initially challenging for some learners
Opens doors to STEM careers	Requires a strong foundation in algebra and geometry
Applicable in various real-world scenarios	Abstract concepts can be difficult to visualize

Best Practices for Mastering Trigonometry

Navigating the world of trigonometry becomes smoother with the right strategies in place. Here are some best practices to guide your learning journey:

1. Build a Strong Foundation: Ensure you have a solid grasp of algebra and geometry concepts, particularly those related to triangles, angles, and ratios.

2. Understand the Unit Circle: The unit circle is a powerful tool for visualizing trigonometric functions and their relationships. Familiarize yourself with its properties and how to use it to find trigonometric values.

3. Practice, Practice, Practice: The key to mastering any mathematical concept is consistent practice. Solve a variety of trigonometry problems, gradually increasing the level of difficulty.

4. Seek Help When Needed: Don't be afraid to ask for help from your teacher, classmates, or online resources if you're struggling with a particular concept.

5. Relate to Real-World Applications: Trigonometry isn't just about abstract formulas. Try to connect the concepts you're learning to real-world applications, which can make the material more engaging and easier to understand.

Frequently Asked Questions about Trigonometry Problems

1. What are the main trigonometric ratios?

The main trigonometric ratios are sine (opposite/hypotenuse), cosine (adjacent/hypotenuse), and tangent (opposite/adjacent).

2. What is the Pythagorean Theorem, and how is it used in trigonometry?

The Pythagorean Theorem states that in a right triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This theorem is used extensively in trigonometry to find missing side lengths of right triangles.

3. What are trigonometric identities, and why are they important?

Trigonometric identities are equations that are true for all values of the variables for which they are defined. They are useful for simplifying trigonometric expressions and solving trigonometric equations.

4. How is trigonometry used in real life?

Trigonometry has numerous applications in fields such as architecture, engineering, physics, navigation, and surveying. For example, architects use trigonometry to calculate roof slopes and structural loads, while engineers use it to design bridges, buildings, and other structures.

5. What are some common mistakes to avoid when solving trigonometry problems?

Some common mistakes include using the wrong trigonometric ratio, forgetting to convert between degrees and radians, and not considering the quadrant of the angle when finding trigonometric values.

6. What are some tips for memorizing the unit circle?

Try to understand the patterns and relationships between the angles and their corresponding trigonometric values rather than simply memorizing the unit circle. You can also use mnemonics or create your own memory aids.

7. How can I improve my trigonometry problem-solving skills?

The best way to improve your skills is through consistent practice. Start with simpler problems and gradually work your way up to more challenging ones.

8. What resources are available for learning trigonometry?

There are countless resources available, including textbooks, online courses, video tutorials, and mobile apps. Khan Academy, Purplemath, and MathPapa are excellent online resources that offer comprehensive trigonometry lessons and practice problems.

Conclusion

Mastering trigonometry might seem like scaling a mathematical mountain, but the view from the top is undoubtedly rewarding. This journey, while potentially challenging, is ultimately about empowering yourself with a versatile toolset applicable across diverse fields. By embracing the principles, practicing diligently, and seeking help when needed, you can conquer even the most daunting trigonometric peaks. Remember, it's not about memorizing formulas but about understanding the relationships and applying the concepts to unravel the mysteries hidden within triangles and beyond. So, embrace the challenge, and unlock the power of trigonometry!

Crafting the perfect hilarious instagram username for guys
The undying allure of online greatest hits radio
Unlocking serenity the power of imagenes de colores pastel